Unlike a cube, a regular octahedron contains points that are above only one of its facets.
The 48 little tetrahedra on the left (six clusters, each containing four pairs sharing an edge) show where these are located
(in an "invisible" octahedron). On the right you see the octahedron with those bits missing.
If you were to make an octahedral die weighted so that the center of gravity was within one of the regions on the left, it would only be able to stand on one face, no matter how carefully you tried to stand in on another face. A cubic die, by contrast, is always stable on every facet, no matter how it may be weighted.
A 16-cell is the four-dimensional analogue of an octahedron. (It's one of the three infinite
families of regular spherical polytopes: the others are the simplexes - like triangles and tetrahedra
- and the measure polytopes - like squares and cubes.) A 16-cell has sixteen tetrahedral facets.As with the octahedron above, a 16-cell has interior points that are above only one facet. This picture shows one facet, with (on the left) the regions adjacent to interior points that aren't above any other facet, and (on the right) the rest of the tetrahedron. This picture is analogous to one triangular face of the picture above.
A 600-cell is a four-dimensional polytope that has 600 (!) tetrahedral facets,
with twenty meeting at each vertex and five around each edge. Its closest three-dimensional analogue is the icosahedron.
This picture shows one facet of a 600-cell. The opaque regions are the parts above some other facet. They consist of an octahedral region above the diametrically opposite facet, and four tetrahedral spikes, over facets adjacent to the opposite facet. The spikes are "stretched" by the angle of projection so that they extend right through the octahedron, in the same way that objects cast long shadows near sunset. The regions that "cast" them are actually the same height as the octahedron.
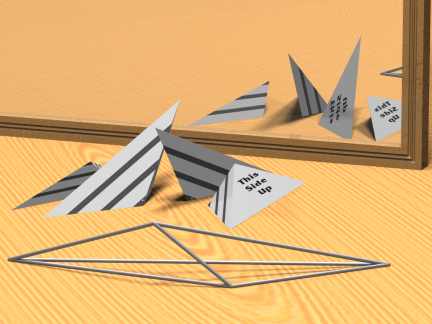This non-regular tetrahedron, first discovered by J.H. Conway, is stable
on only one facet when suitably weighted. It is shown, from back to front, in the stages of falling
"through" its facets, with a wireframe model in the foreground. The mirror is to permit another viewpoint.
The center of gravity must be in a very small region near the apex (shown as a light-colored dot, just visible on the second copy.) Making a model of this that really works is hard, even with a lightweight skeleton and a lead weight! Usually it ends up too floppy to really work properly.
This enneadecahedron (nineteen-faceted polyhedron) is only stable on one of its facets
if it is constructed of material of uniform density. Its cross section consists of two discrete approximations
to an equiangular spiral, as shown by the "tree rings" on the ends (which are around an axis through the center of mass).
The ends are sloped, which serves two purposes: firstly, it moves the center of gravity further towards one side,
and secondly it makes the body unstable on those facets as well. It was discovered by John Conway and Richard Guy
in 1969. It is shown (at right) about to start falling from one of its ends, and (at left) in a state of rest.
It is not known whether this polyhedron has the minimal number of faces for one with such a property in three-dimensional space - it seems probable but not certain. It is known that a certain irregular simplex of uniform density in 10-dimensional space is stable on only one facet; this has 11 facets. An interesting open question - can 10 facets be achieved in some dimension between 3 and 10?
Another question, about which practically nothing is known, involves polytopes whose mass is uniformly distributed over their n-skeleta. If the mass of a simplex is equally distributed among its vertices then its center of gravity is the same as if it is made of a solid homogeneous material; but if its mass is concentrated in an n-skeleton of uniform density [imagine a tetrahedron with edges made of wire, or hollow with cardboard faces] the center of gravity will generally be different.
|
TRIVIA QUESTION What has all of this got to do with films such as "The Birds" and "I  Huckabees"?Answer: |
